Solutions to SQA examination

1994 Higher Grade Physics

Paper II Solutions

1.a.i.	At the maximum height the long jumpers vertical velocity(V_v) is 0m/s.
	
	v_v = 0m/s
	S_v = 0.86m
	a = -9.8m/s/s
	u_v = ?

	To find u use : v² = u² + 2as

	0² = u² + 2x(-9.8)x0.86
	u² = 16.856(m/s)² 
	u = 4.1m/s		  

	Note: 	The positive square root is taken because 
		the motion is in the upwards direction.

  a.ii.	The total time in the air for the vertical jump in part a.i. will be
	the same as that for the actual jump if the vertical component of velocity 
	is the same.
	
	The total displacement for the jump in part a.i. is 0m.

	s = 0m
	a = -9.8m/s/s
	u = 4.1m/s
	t = ?

	To solve for t use: s = ut + 1/2(at²)
	
	0 = ut + 1/2(at²)....divide by t
	0 = u + (at)/2
	t = -2u/a
	t = (-2x4.1)/-9.8
	t = 0.84s

	v_H = s_H/t
	v_H = 7.8/0.84
	v_H = 9.3m/s

  b.	With a maximum height of less than 0.86m the time in the air would
	be less than that 0.84s. This means that the horizontal distance
	must be covered in a shorter time. This can only be achieved if
	the horizontal velocity is greater than 9.3m/s.

2.a.i.	


  a.ii.	m = 500kg
	g = 9.8N/kg
	W = ?

	W = mg
	W = 500x9.8
	W = 4900N

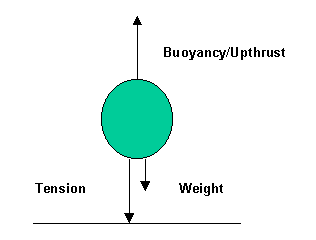
	With no rope in position there is no tension force(T). This means
	that the unbalance force causing the acceleration is the difference
	between the buoyancy force(B) and the weight(W).

	F_un = B - W 
	F_un = ma

	ma = B - W
	B = ma + W
	B = 500x1.5 + 4900
	B = 5650N

  a.iii.Before the release the upward force must balance the downward forces.

	B = W + T
	T = B - W
	T = 5650 - 4900
	T = 750N
 
  b.	

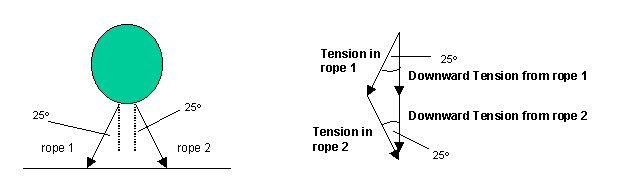	Each rope contributes half of the downward component of the tension.

	Downward tension from rope 1 = 375N
	Downward tension from rope 2 = 375N

	To calculate the tension in each rope use trigonometry.

	375/T_{rope 1} = cos25^o
	T_{rope 1} = 375/cos25^o
	T_{rope 1} = 413.8N

	375/T_{rope 2} = cos25^o
	T_{rope 2} = 375/cos25^o
	T_{rope 2} = 413.8N

4.a.i.	Use Boyle's law to solve this problem.

	P₁ = 1.76x10⁵Pa
	V₁ = 750cm³			
	P₂ = ?
	V₂ = 900cm³

	P₁V₁ = P₂V₂
	P₂ = P₁V₁/V₂
	P₂ = 1.76x10⁵x750/900
	P₂ = 1.47x10⁵Pa

  a.ii.	F = PA
	F = 1.47x10⁵x5x10^-3
	F = 735N

  b.	The gas inside the rocket exerts the forces shown in the diagram.

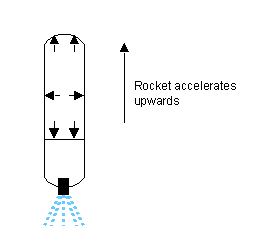
The lateral forces cancel.
The downward force accelerates the water out of the rocket.
The upward force accelerates the rocket.


4.a.	Ek = 1/2(mv²)

	Collision with polyurethane block.
	Ek = 1/2(0.5x0.33²)
	Ek = 0.027J

	Collision with rubber band.
	Ek = 1/2(0.5x0.43²)
	Ek = 0.046J

  b.	In elastic collisions kinetic energy is conserved.
	In the collision with the metal spring the least amount of kinetic 
	energy is lost and is therefore the most like an elastic collision.

  c.	To propel the vehicle with the same initial speed the force providing
	the impulse must be equal in each experiment. This could be achieved
	if the impulse was provided by a stretched elastic band and ensuring
	the band was pulled back by the same amount each time. 
	

  d.i.	F = (mv-mu)/t_c

	m = 0.5kg
	t_c = contact time = 0.4s
	u = -0.55m/s
	v = 0.35m/s

	F = 0.5[0.35-(-0.55)]/0.4
	F = 0.5x0.9/.4
	F = 1.125N

  d.ii.	The vehicle accelerates to the right when in contact with the block.
	This is because the direction of the force causing the acceleration 
	is towards the right and the acceleration must be in the same direction.

5.a.i.	V_supply = V_x + V_y
	V_x = V_supply - V_y
	V_x = 10 - 6
	V_x = 4V

	I_x = V_x/R_x
	I_x = 4/1200
	I_x = 0.0033A

	I_x = I_y = 0.0033A

	V_y = I_yR_y
	R_y = V_y/I_y
	R_y = 6/0.0033
	R_y = 1800W = 1.8kW

5.a.ii.	The voltage across each resistor in a potential divider circuit
	is proportional to the value of its resistance. When resistor Z 
	is connected in parallel with resistor Y a parallel network, with
	a lower resistance than resistor Y alone, is created. The voltage across 
	this network, and each resistor in the network, is therefore less than 
	the voltage across Y alone.

5.a.iii.1/R_p = 1/R_Y + 1/R_Z
	1/R_p = 1/1.8 + 1/4.7
	1/R_p = 0.5555 + 0.2128 
	1/R_p = 0.7683
	R_p = 1.3kW

	V_{R_p} = [R_p/(R_X+R_p)]V_supply

	V_{R_p} = [1.3/(1.3+1.2)]10
	V_{R_p} = 0.52x10
	V_{R_p} = 5.2V

5.b.i. R_A/R_B = R_C/R_D

b.ii.

Resistance of A/W	Resistance of B/W	Voltmeter reading/mV
120	120	0
121	120	-21
121	121	0
121	122	+21
121	119	-42

	The values in the table are worked out using the fact that the 
	voltage reading is proportional to the out of balance resistance. 

6.a.i.	Q = Ixt

	Q = ?
	I = 0.5A
	t = 1h = 3600s

	Q = 0.5x3600
	Q = 1800C

6.a.ii.	E = Pt
	P = IV
	=>E=ItV
	E = 0.5x3600x1.2
	E = 2160J

	OR

	E = QV (same answer)

  b.i. 	The emf is the energy the cell supplies to each coulomb of charge 
	passing through it.

  b.ii.	The emf can be found by projecting the graph line back until it 
	cuts the voltage axis.

	emf = 1.4V

	The internal resistance is equal to the negative of the gradient
	of the line given.

	m = (y₁-y₂)/(x₁-x₂)
	m = (1.0-0.6)/(1.0-2.0)
	m = 0.4/-0.1
	m = -4

	r = 4W

	To justify the above consider:

	y = mx + c  ...1
	V = mI + c  ...2
	V_tpd= E - Ir   ...3
	V_tpd= -Ir + E  ...4

	From equation (3) 
	When I = 0A :V_tpd=emf
	
	Comparing (2) and (4)
	m = -r

7.a.i.	The light from the window will result in the solar cell producing
	a small voltage that is amplified to produce a non zero V_out.

  a.ii.	V_out/V_in = R_feedback/R₁
	V_in = -V_out(R₁/R_feedback)
	V_in = -(-1.75)(15/120)
	V_in = 0.219V

  b.i.	The differential amplifier amplifies the difference between V₁ and V₂. 
	When V₁ is adjusted to equal V₂ there is no difference between 
	the input voltages and the output voltage will be zero.

  b.ii.	V_out = R_f/R₁(V₂-V₁)
	1.5 = 220/4.7(V₂-0.219) 
	1.5 = 46.81(V₂-0.219)
	0.032 = V₂-0.219
	V₂ = 0.251V

8.a.	T = 4x5ms
	T = 20ms

	f = 1/T
	f = 1/20x10^-3
	f = 50Hz

  b.i.	The value of resistor R₂ was increased. This conclusion is drawn
	because the rate at which the capacitor discharges has decreased.

  b.ii.	Q = CDV

	DV = V_initial-V_final
	DV = 8V - 2V = 6V
	
	Q = 2.2x10^-6x6
	Q = 1.32x10^-5C

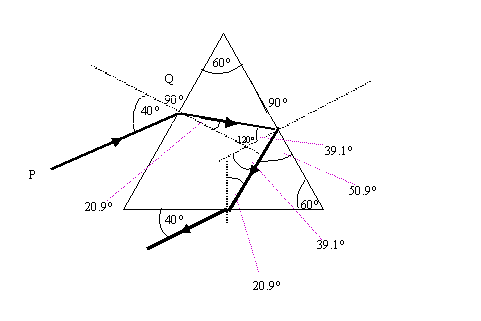
9.a.i.	n_plastic = sinq_air/sinq_plastic
	n_plastic = sin40^o/sin30^o
	n_plastic = 0.633/0.5
	n_plastic = 1.29

  a.ii. 


  b.i.	q_critical = sin^-1(1/n)
	q_critical = sin^-1(1/1.8)
	q_critical = sin^-1(0.555)
	q_critical = 33.7^o

  b.ii.	n_{borate glass} = sinq_air/sinq_{borate glass}
	sinq_{borate glass} = sinq_air/n_{borate glass}
	sinq_{borate glass} = sin40^o/1.8
	sinq_{borate glass} = 0.0.643/1.8
	sinq_{borate glass} = 0.357
	q_{borate glass} = 20.9^o
	


10.a.i.	Electrons are moving from a high to low energy level within the atom.
	A photon of light is emitted when this happens.

  a.ii.	l_{sodium yellow} = 589nm
	E_photon = hf
	E_photon = hc/l
	E_photon = 6.63x10^-34x3x10⁸/589x10^-9
	E_photon = 3.38x10^-19J

	The photon energy and the energy difference are equal.
	E_difference = 3.38x10^-19J
	
  b.i.	Photons emitted from the sodium lamp and passing through the flame containing
 	vaporised sodium will be absorbed by sodium electrons. This means that sodium 
	light passing through the flame will be reduced in intensity and produce a dark
 	shadow behind the flame.

  b.ii. There is no energy gap in cadmium with the same energy as a photon emitted
	from the sodium lamp. Therefore, no absorption will take place and there will
	be no shadow region.

11.a.	The activity(A), measured in becquerels(bq), is a measure of the number
	of disintegrations(N) per second.

	A = N/t
	N = At
	N = 300x10⁶x60
	N = 18x10⁹

  b.	H/t = 16mSv/h	(at a distance of 1m)
	H = DQ
	H/t = (D/t)xQ
	16mSv/h = (50mGy/t)x1
	t = 50mGy/16mSv/h
 	t = 3.125h

  c.i.	
	


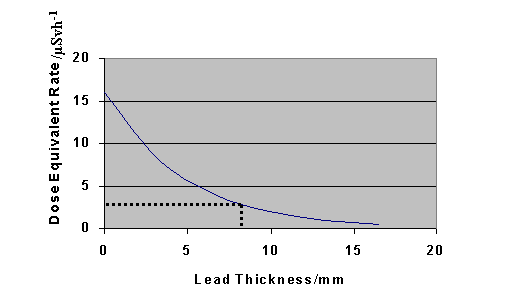  c.ii.	The graph indicates that the required lead thickness is 8.8mm.
 
  d.	The polystyrene packaging increases the distance between the porters 
	and the source. As the intensity at a distance(d) from the source is 
	inversely proportional to the square of the distance the dose equivalent
	rate for the porters will be less.

12.a.	Mean(L) = Total/Number of readings
	Mean(L) = (2.402+2.399+2.412+2.408+2.388+2.383+2.415)/7
	Mean(L) = 16.807/7
	Mean(L) = 2.401m

	Random Error(L) = Range/Number of readings
	Random Error(L) = (Max-Min)/N
	Random Error(L) = (2.415-2.383)/7
	Random Error(L) = 0.032/7
	Random Error(L) = 0.005m

  b.	Percentage error in L = [Random Error(L)/Mean(L)]x100
	Percentage error in L = [0.005/2.401]x100
	Percentage error in L = 0.21%
 
	Percentage error in x = [Random Error(x)/Mean(x)]x100
	Percentage error in x = [1/91]x100
	Percentage error in x = 1.1%

	The measurement with the largest percentage error is x.

  c.	l = dsinq
	l = dx/L
	l = 1.693x10^-591x10^-3/2.401
	l = 641.66nm

	The percentage error in l can be taken to
	be equal to the percentage error in x, as this is the largest individual
	error.

	1.1% of 641.66nm = (1.1/100)x641.66 = 7.06nm
	l = (641.66 +- 7.06)nm
  
  d.	Increasing the distance between the grating		
	and the screen reduces the percentage error 
	of L and, more significantly, x.



END OF QUESTION PAPER


Return to past paper index page.